
Mathematical Software. Mathematical Research. Mathematical Education. Tvalx Products.
Gamma function is the first among special functions. Many other special functions are calculated through Gamma function. Let's explore Gamma function using Math Center Level2.
Using Spouge approximation we get graph (if you own Math Center Level 2 include text ((x-1+10)^(x-1+0.5))*(e^(-x+1-10))*((2π)^(0.5)+Σ(1;10-1;(((-1)^(k-1)/(k-1)!)*(-k+10)^(k-0.5)*e^(-k+10))/(x-1+k))) into Edit window) :

The same in black palette :
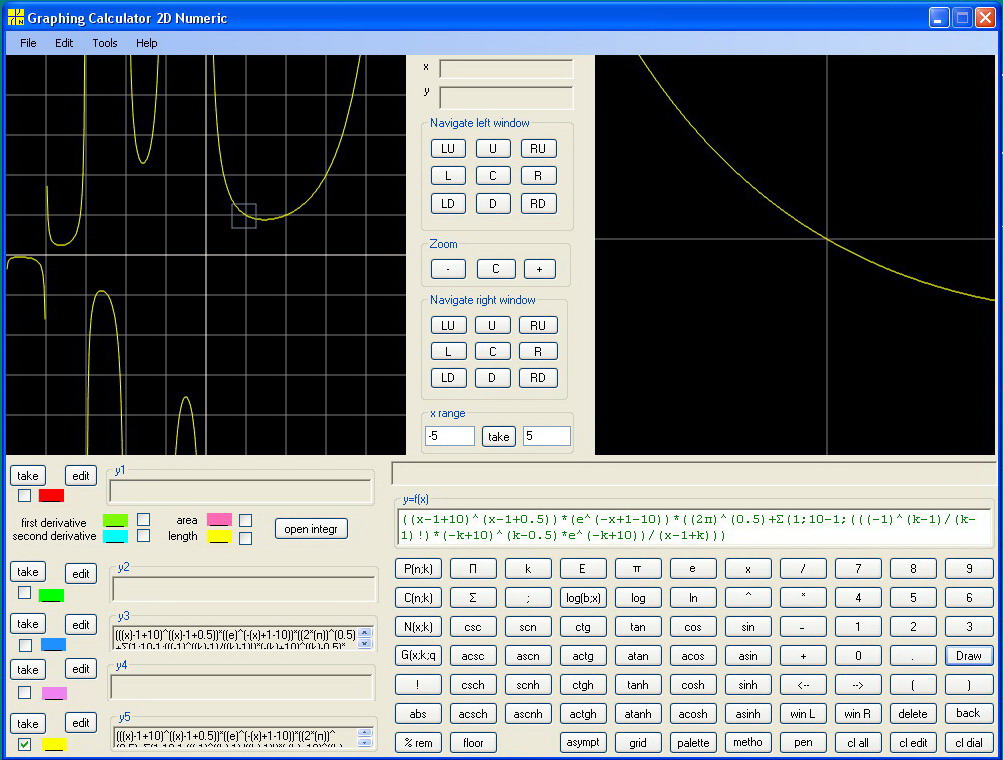
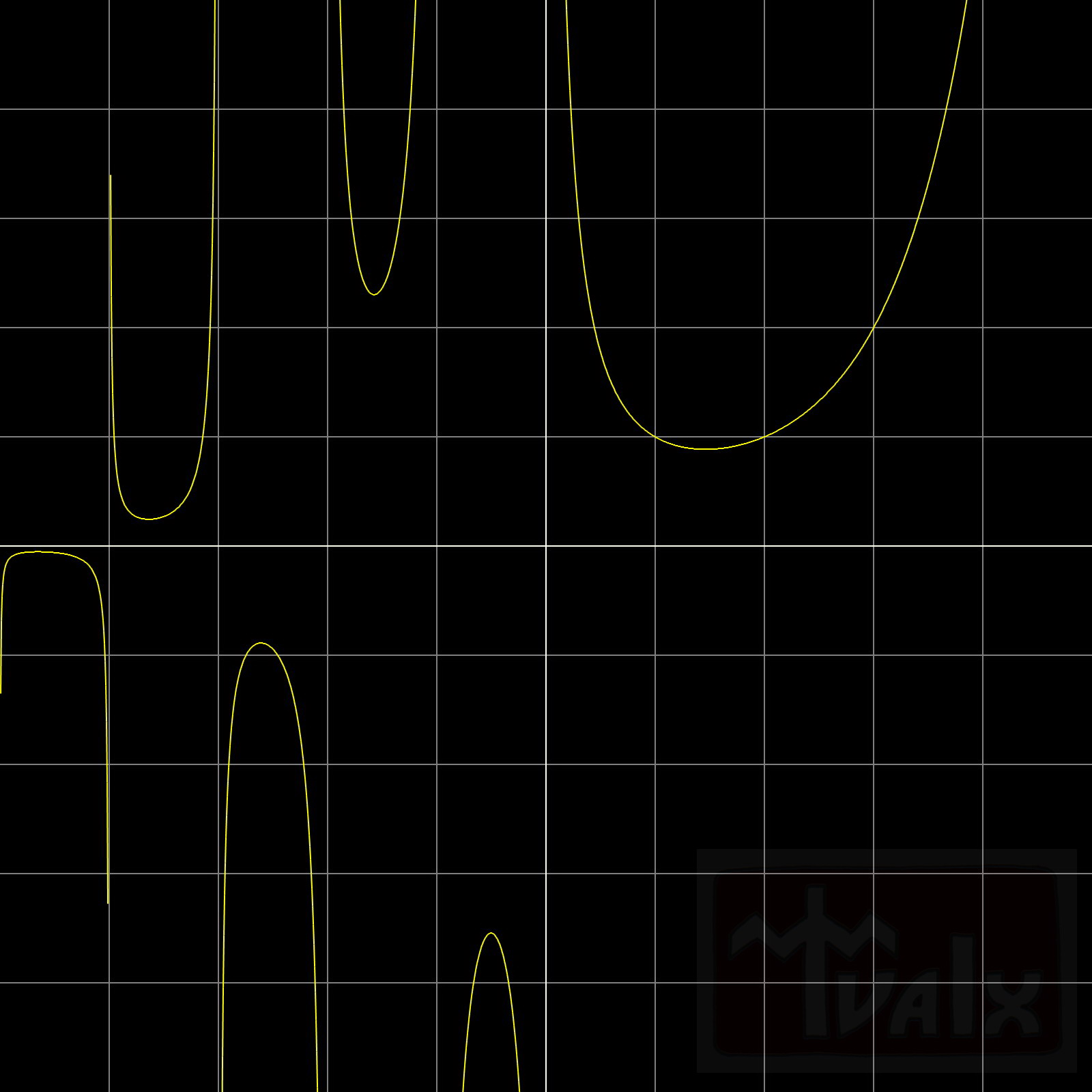
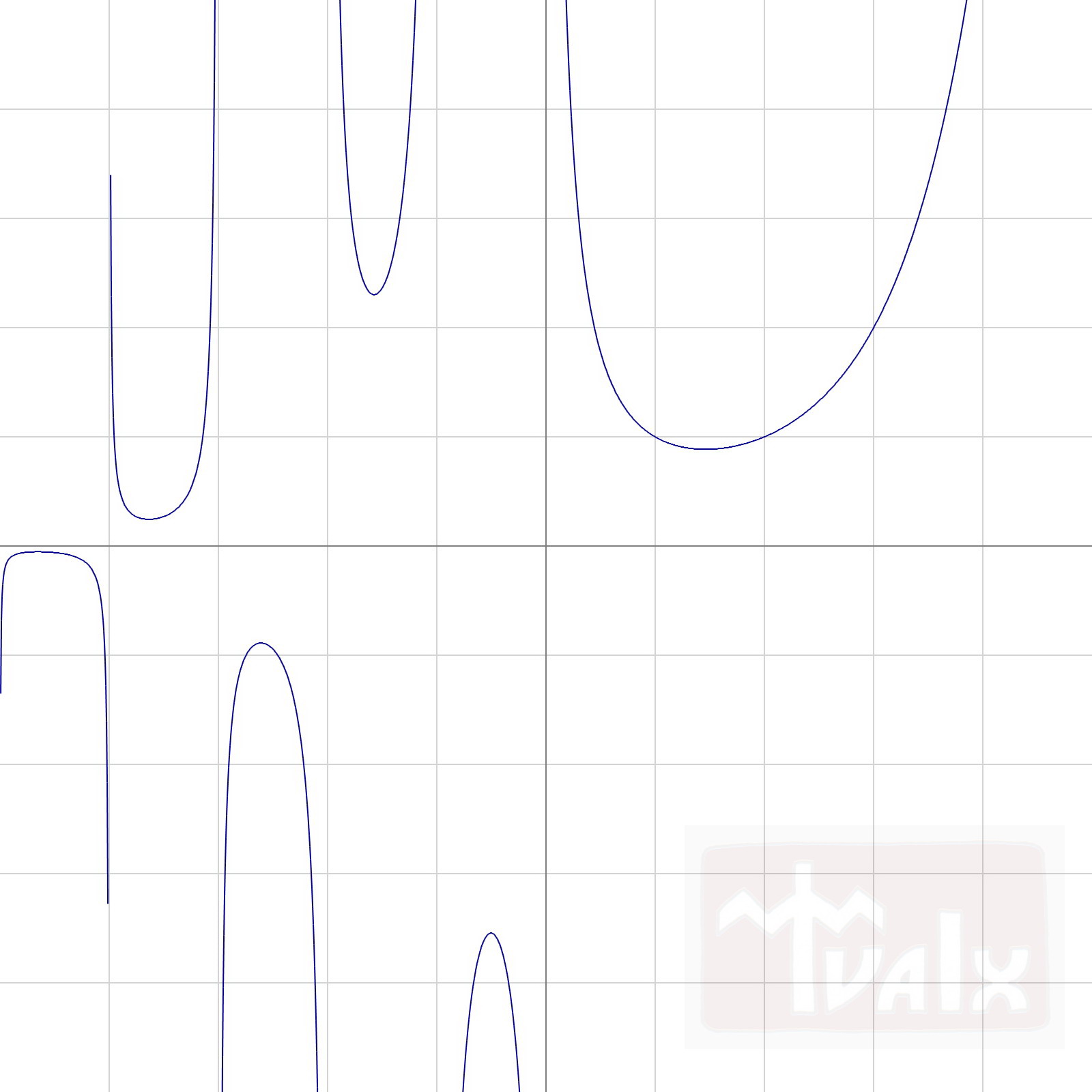
The domain of Gamma function is the positive real half-line and negative half-line with excluded zero and negative integers. The range of Gamma function is entire real line. As a complex function Gamma function has value Infinity (has a pole) at non-positive integer x.
Observing general shape of Gamma function we can say that numerical approximation methods will have very good accuracy on intervals (0.5, 3), and near -n+0.5, especially for big n. Accuracy will be good for x>2. Accuracy will be low near negative integers, especially around 0, -1, -2 .
© 2008 Tvalx
