Mathematical Software. Mathematical Research. Mathematical Education. Tvalx Products.
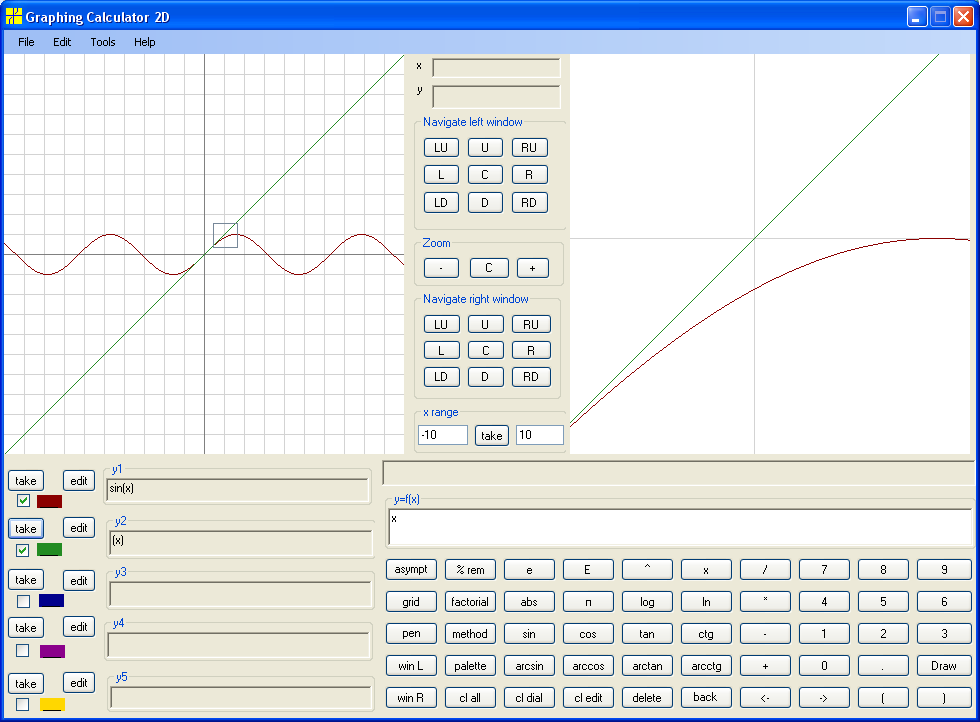
Let's start with Sine y = sin(x) :

Note that near origin graph of y=sin(x) is close to graph of y=x. We see that Sine is a periodic function. Sine has a (single) period 2π, that is sin(x)=sin(x+2π) for any x. The domain of Sine is entire real line and the range is interval [-1, 1] . By convention brackets are used for denoting interval when endpoints are included into interval.
Next is Cosine y = cos(x) :
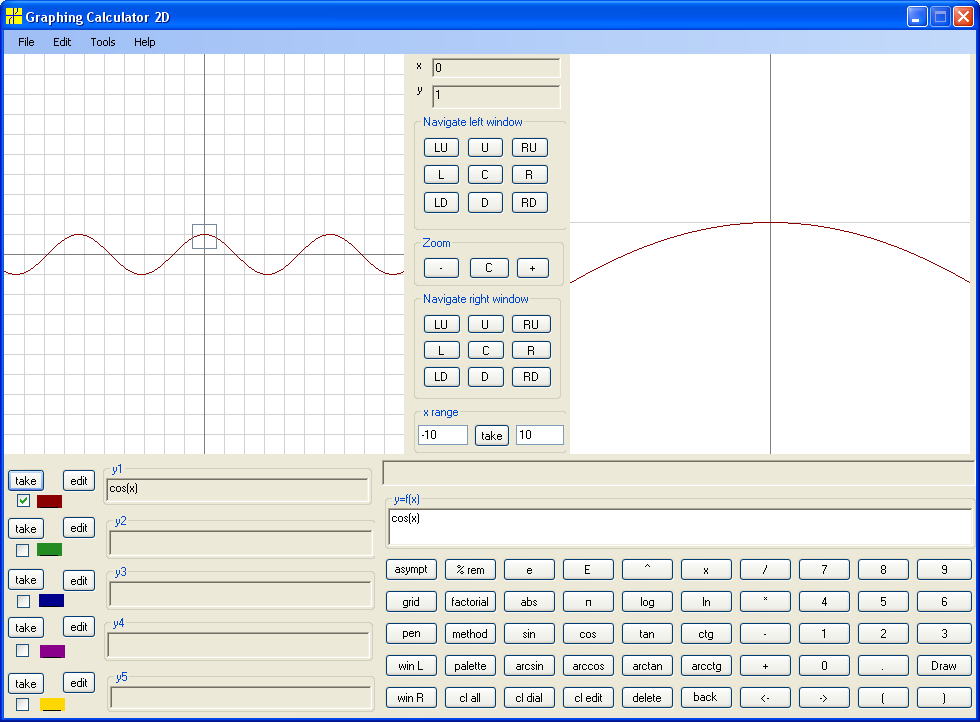
At x=0 the graph of y=cos(x) is close to graph y=1. Cosine is also a periodic functions with period 2π. Note that graph of cos(x) coincides with graph of sin(x) if we shift it by -π/2+2πn :
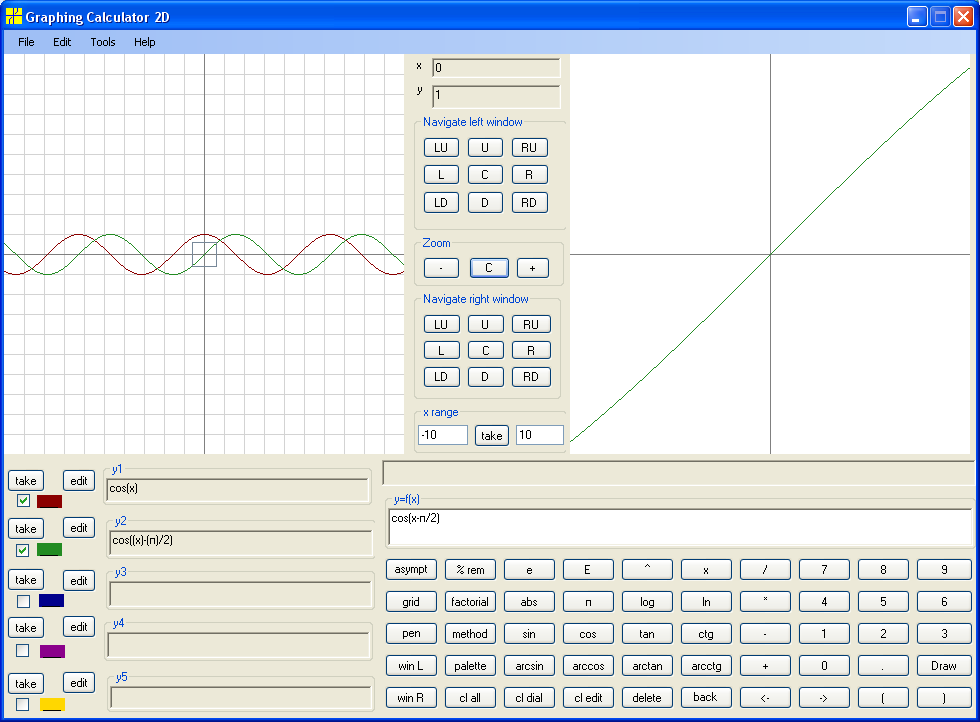
Note that for shifting graph to the left by s units we add s to the argument x: f(x+s). For shifting graph to the right we subtract s units from argument: f(x-s) .
Tangent by definition is sin(x)/cos(x). When cos(x)=0 (at x=π/2+2πn) the expression sin(x)/cos(x) is not defined. Thus tan(x) is not defined at x=π/2+2πn for each integer n.
Let's look at the graph of y = tan(x) :

From the left to each "critical point" x=π/2+2πn y goes to plus infinity (that is goes up) and from the right y goes to minus infinity (that is goes down) .
Cotangent by definition is 1/tan(x) = cos(x) / sin(x) . Thus where Tangent has zero Cotangent has infinity and where Tangent has infinity Cotangent has zero:
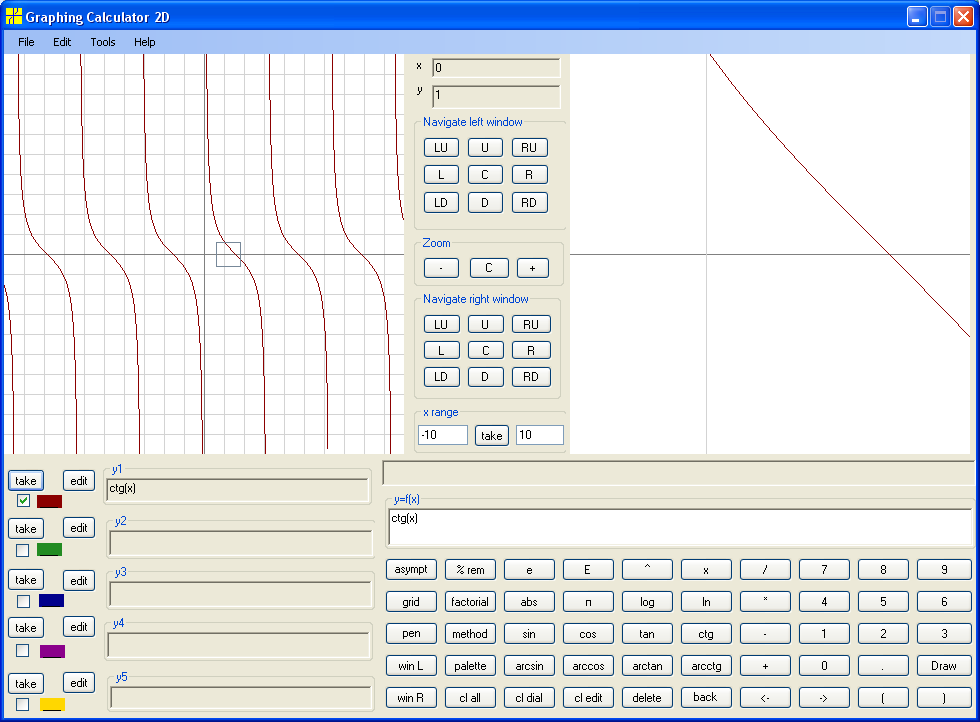
Both Tangent and Cotangent have period 2π.
In situations when graph of function goes to infinity at some critical point, a vertical line passing through the critical point is called a vertical asymptote. In general, an asymptote of function is a (straight) line which graph of function approaches "infinitely close". Pressing button "asympt" we draw asymptotes for Tangent and Cotangent:
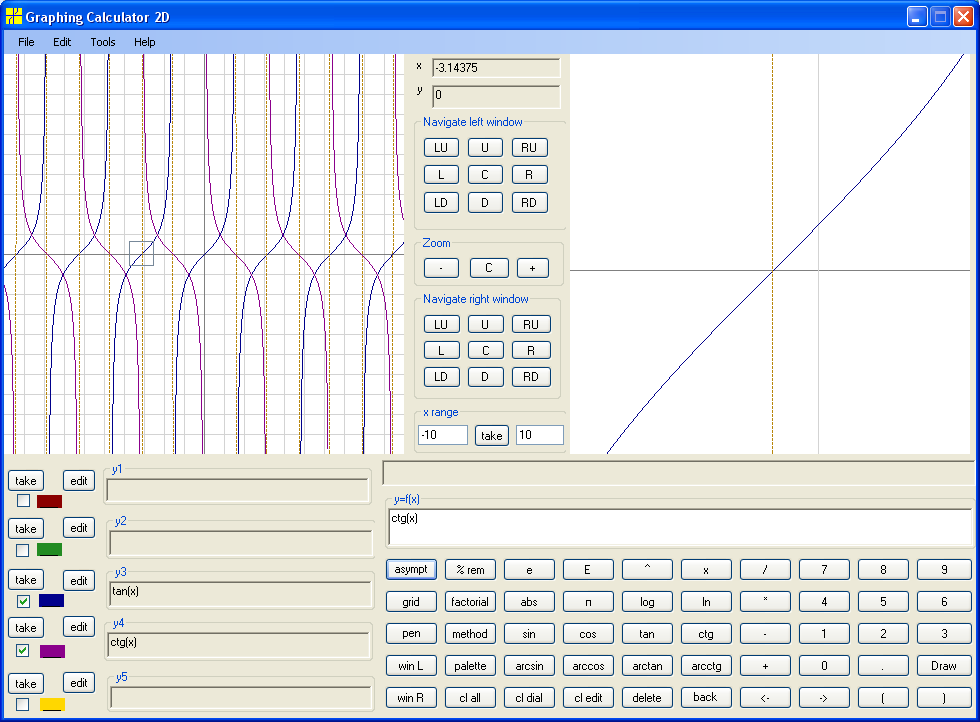
By clicking on intersection of dark blue line and dark gold dashed line in right window we get coordinates of the point where Cotangent is zero and tangent is infinity. that is x=π/2. In general x=π/2+2πn. There different notations used for Tangent: tan, tng, tg - and for Cotangent: cot, ctg, ctn .
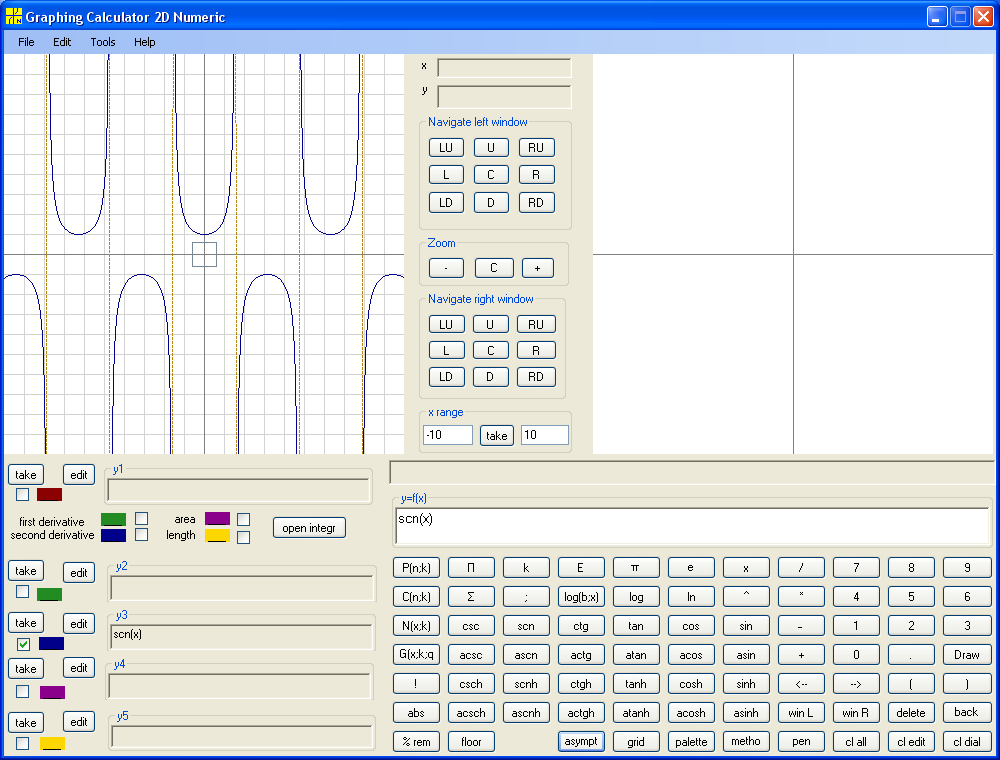
Secant and Cosecant are less known trigonometric functions. They are present in Math Center Level 2 . There are different notations used for Secant: sec, sct - and for Cosecant: csc, cst, cosec.
By definition sec(x)=1/cos(x) and csc=1/sin(x). Thus Secant has vertical asymptotes where Cosine has zeroes and Cosecant has vertical asymptotes where Sine has zeroes.
Let's look at y = sec(x):
Now look at y = csc(x) :
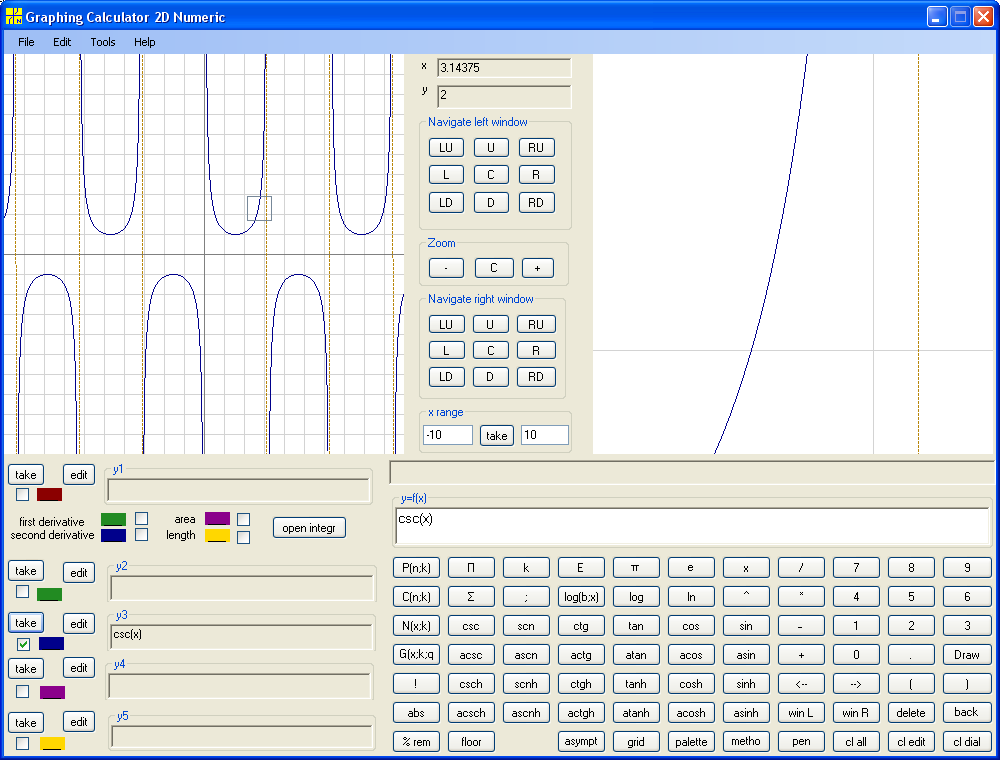
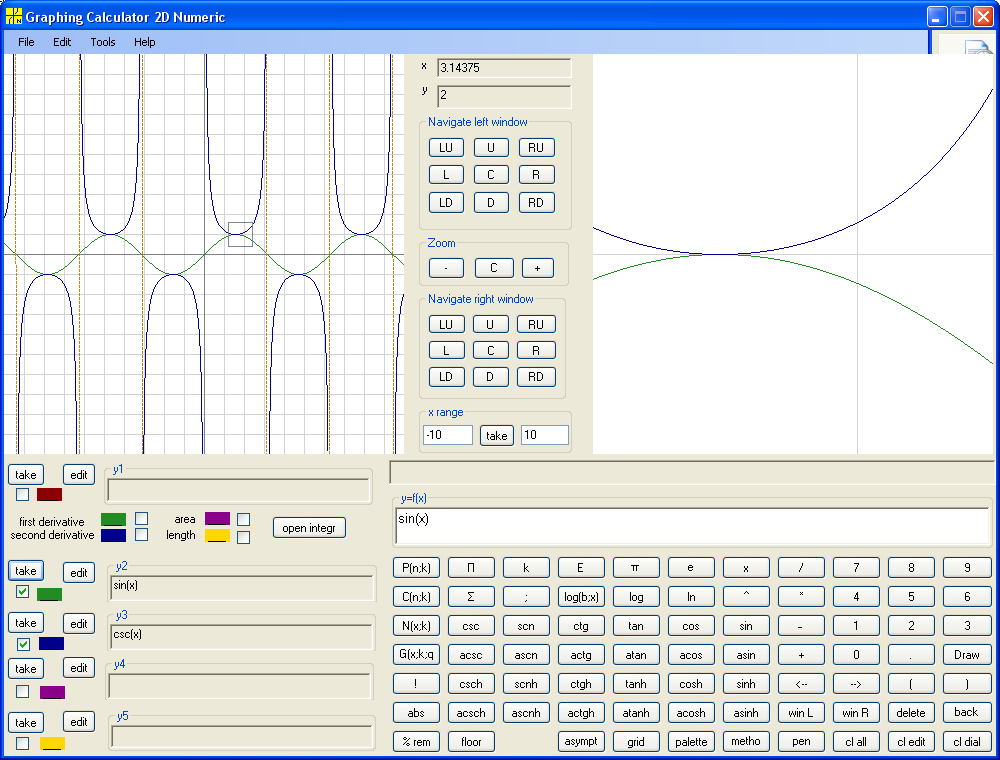
Secant and Cosecant have period 2π.
Let's recall trigonometric equalities and explore them with help Graphing Calculator 2D from Math Center Level 1 and Math Center Level 2 .
Recall sin2(x) + cos2(x) = 1 . On the picture below red graph is for sin, green for cos, blue for sin2, purple for cos2, and yellow is for sin2 + cos2 :

Recall sin(2x)=2sin(x)cos(x) :
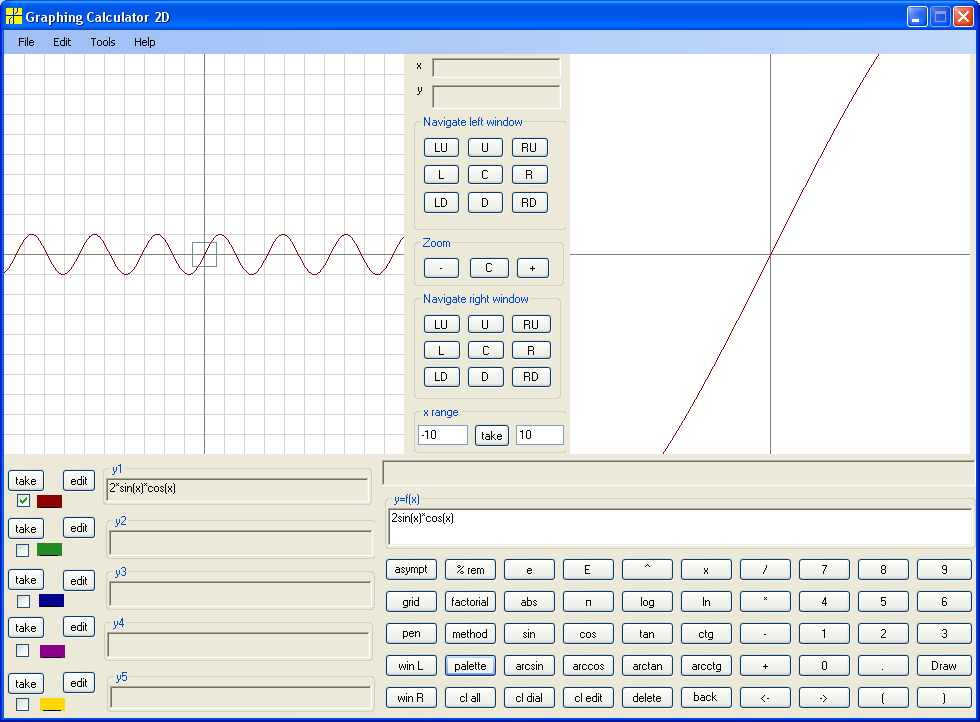
More rear identity sin(3x)=3sin(x)-4sin3(x) :
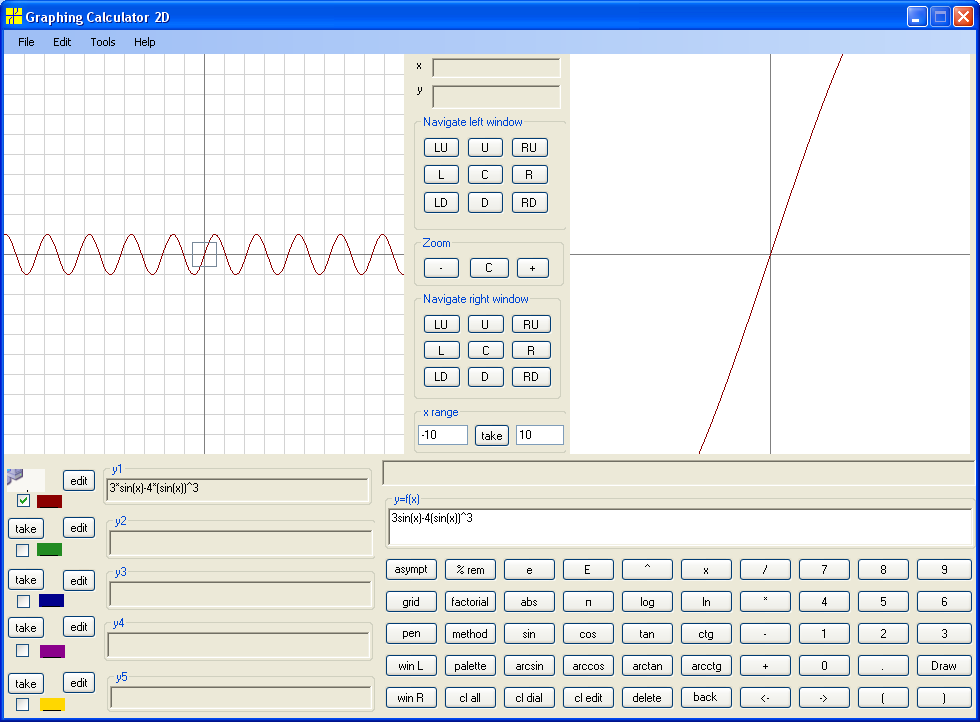
© 2008 Tvalx
